This page is on a topic that I have wondered about. I would appreciate any feedback that you might be able to provide. Especially errors in concept or calculation. Please send an email to jdj@mcanv.com if you would care to comment.

I am going to begin this page by trying to get a grip on the entropy of the very early universe. If you have not digested the ideas expressed on the prior page you should review that before you take on this one. There is currently a popular TV sitcom called "The Big Bang Theory" where the opening theme song declares the universe began in a hot, dense state. They fail to mention that it was also a thermalized state, where thermalized means that it was uniform in temperature throughout. There is abundant physical evidence (see "The Road to Reality" 27.13) that this was the case. Given what I have told you so far about entropy, you would be justified in concluding that this hot, dense, thermal state was a case of maximum entropy. Clearly that can not be the case since the universe has been rolling downhill ever since, gathering entropy as it goes in accordance with the second law of thermodynamics.

What I have failed to take into account in my explanation of entropy so far is the effect of gravity. My excuse is that gravity is such a weak force that in ordinary circumstances, for boxes of gas molecules or the heat engines we deal with here on earth it is the interactions among bits of matter and radiation that dominate. Gravity plays essentially no role. For the box of gas molecules we found the uniform distribution of the molecules to be the high entropy state. We would have to do considerable work on the system to push all of those molecules into a tiny corner of the box. The 'box mostly empty' distribution is clearly unstable.

In the universe as a whole, the effect of gravity clearly can not be neglected. So instead of a human sized box of gas molecules lets consider a galaxy sized box of uniformly distributed dust. In this case the uniform distribution is unstable. Tiny disturbances to the uniformity result in the dust tending to clump together under the influence of gravity and the clumps grow as they attract more dust and perhaps some of the clumps join to make bigger clumps. Now we would have to do a huge amount of work to pull the clumps all apart and restore the uniform dust state. Think of the work involved in just separating the anchor of your boat from the bottom of the bay by 40 feet or so. Thus a uniform dust is a low entropy state and clumped matter is a higher entropy state.

Some time back, about three to four hundred thousand years after the big bang, radiation and matter began to lead separate lives so I will take that timeframe to try to find the volume of the universal phase space taken up by the matter and radiation. That should give us a conservative estimate of the phase space volume at earlier times closer to the big bang. At the decoupling of radiation and matter it is estimated that almost all the mass of matter was carried by 10⁸⁰ particles called baryons, basically protons and neutrons. Also at that time there were roughly 10⁸ photons of radiation for every baryon. It turns out that the contribution of the radiation to the entropy of the universe completely overwhelms the contribution from the matter. In Planck units the sum of the matter and radiation entropy at the decoupling is roughly the number of photons per baryon times the number of baryons = 10⁸X10⁸⁰ = 10⁸⁸. Remember that the entropy is the logarithm of the phase space volume so the phase space volume is roughly 10¹⁰⁸⁸. In addition to the baryons there seems to be considerable dark matter in the universe, matter that is evident only from its gravitational effect. Let's jack up the estimate of the phase space volume at the big bang by a factor of 100 to accomodate any dark matter effect, yielding 10¹⁰⁹⁰

Pause for a moment and think about the magnitude of that number that I am going to take to be the volume of the universal phase space occupied by the big bang. Written out it would be 1 followed by 10⁹⁰ zeros. If a zero was a tenth of an inch wide 10⁹⁰ of them would span 10⁸⁹ inches or 2.5X10⁸⁹ meters. For comparison the diameter of the observable universe is 8.8X10²⁶ meters. There would not be room in the entire observable universe for that many printed zeros.

So we have one of the two numbers I proposed we go after, back at the end of the prior page. The other was the total entropy available to the universe. It turns out that there is a way to access this number that involves consideration of black holes. These are regions where the gravity is so strong that nothing, including light, that enters the region ever escapes. One theory of the end of the universe suggests that black holes continue to suck in matter and radiation, and even other black holes until the universe as we know it ends up in a congealing mess of black holes. In fact a black hole of about 3X10⁶ times the mass of our sun exists at the center of our galaxy. Even now all the stars of the galaxy are circling the drain, so to speak, on their way to absorption in this black hole.

It is not obvious without some explanation that a black hole represents a very large entropy configuration. To quote from "The Road to Reality",
"The relentless nature of a black hole, as it sweeps up all kinds of material-which could have an immense amount of detailed structure-converting it into a single configuration describable by only ten parameters (these being a, m, the direction of the spin axis, the position of the mass centre, and its 3-velocity) is a powerful manifestation of the second law. These ten parameters are all that are needed for an adequate macroscopic characterization of the final state. Although a black hole does not look like ordinary matter in thermal equilibrium, it shares with it the key property that huge numbers of microscopically distinct states lead to something which can be described by very few parameters. For this reason, the corresponding phase-space coarse-graining box is indeed enormous, and black holes, consequently, have enormous entropies."

Stephen Hawking and Jacob Bekenstein have developed a formula to calculate the entropy of a black hole in terms of the horizon surface area. By applying this formula to the estimated black hole population of the universe just before the cannibalization among the holes gets out of hand we can get a reasonable estimate of the total entropy available to the universe. I am not about to replicate the calculations here but the answer in the same units that we used for the big bang entropy is about 10¹²⁴. This leads to a total phase space volume of the universe of 10¹⁰¹²⁴.

How are we to compare the phase space volume at the big bang with the total available to the universe. Let's call the volume of the big bang macrostate B and the volume of the total universe U. Then we can set up a proportion B:U as 1:X, read B is to U as 1 is to X. To find X we just divide both sides of the first proportion by B. Thus X=U/B

Hopefully there are some readers out there who doubt my arithmetic. Here is a quick way to check. Open the calculator that comes with your computer, click on the "View" menu and select "Scientific" then click on the following sequence of keys: 1, Exp, 124, -, 1, Exp, 90, =.

At this point I differ from Professor Penrose's interpretation of the state of affairs we have uncovered on this page. The following quote from "The Road to Reality"28.7 sets forth the Penrose position.

"There was indeed something very special about how the universe started off. It seems to me that there are two possible routes to addressing this question. The difference between the two is a matter of scientific attitude. We might take the position that the initial choice was an 'act of God' (rather like that fancifully illustrated in Fig. 27.21). or we might seek some scientific/mathematical theory to explain the extraordinarily special nature of the Big Bang. My own strong inclination is certainly to try to see how far we can get with the second possibility. We have become used to mathematical laws-laws of extraordinary precision-controlling the physical behavior of the world. It appears that we again require something of exceptional precision, a law that determines the very nature of the Big Bang. But the Big Bang is a spacetime singularity, and our present-day theories are not able to handle this kind of thing. Our expectations, however, are that what is required is some appropriate form of quantum gravity, where the rules of general relativity, of quantum mechanics, and perhaps also of some other unknown physical ingredients, must come together appropriately."

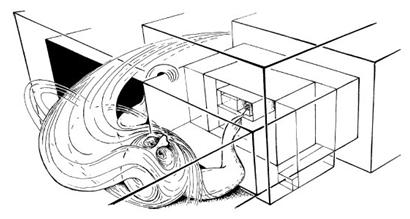
Fig. 27.21 Creation of the universe: a fanciful description! The Creator's pin has to find a tiny box, just 1 part in 1010124 of the entire phase-space volume, in order to create a universe with as special a Big Bang as that we actually find.

It seems to me that Professor Penrose in considering his "act of God" possibility has neglected the enormous power of intelligence in getting improbable results, sort of reducing God to assembling his puzzle by tossing the pieces, all at once, in such a way that it lands assembled. Both of the options offered above are articles of faith, either in a Creator or the power of science. Neither has been conclusively demonstrated. I would not be surprised to find that Professor Penrose's first possibility is actually the case but if the second possibility should prove fruitful, I am willing to wait for the next unanswered question to bring us face to face with the first possibility again.